

So, we found out that the number of circles each passing through three of the given 7 points is equal to the number of all different triples composed of the givenħ points. Thus, the number of different triples composed of 7 points is equal to = 35.
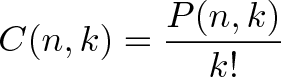
In this way you count each triple 2*3 = 6 times according to the number of permutations of points inside the triple. This gives you 6 independent choices.įinally, you can select any of 5 remaining points as the third point of the triple. Then you can select any of 6 remaining points as the second point of the triple. You can select any of 7 points as the first point of the triple. Now you can easily calculate the number of triples. The order of the points inside the triple does not matter while counting the triples because the permutations of points inside the triple gives the same circle. Therefore, the number of different circles each passing through three of the given 7 points is equal exactly to the number of different triples composed of the givenħ points. Thus, any three points of the given 7 points have the unique circle passing through these three points, and any such a circle corresponds to the unique triple Thus, any triple of the given 7 points has the unique circle passing through these three points.įrom the other side, the different triples of the given 7 points have different respective circumferential circles because no four of the given points are on the same circle. This circle is uniquely defined for any three points in the plane that are not in a same straight line.
Permutation combinations p uplet how to#
How to construct the circle circumferential to the triangle: the intersection of the perpendicular bisectors to the triangle sides is the center of the circle. It is described in the lesson Perpendicular bisectors of a triangle sides are concurrent in this site To the triangle built on these three points as its vertices. How many are there different circles each passing through three of the given points?įirst, for any three points in a plane that are not on the same straight line there is the circle passing through these three points. In turn, this number is equal to = 21.Įxample 2You are given 7 points in a plane such that neither three of them are on the same straight line nor four of them are on the same circle. So, we found out that the number of straight lines each passing through two of the given 7 points is equal to the number of all different pairs composed of the givenħ points.

Thus the number of different pairs composed of the given 7 points is equal to = 21. Permutation of the points inside the pair still remains the pair the same. In this way you count each pair twice because Then you can select any of six remaining points as the second point in the pair. You can select any of seven points as the first point in the pair. Now you can easily calculate the number of pairs. The order of the points inside the pair does not matter while counting the pairs because the permutations of points inside the pair gives the same straight line. This means that the number of all the straight lines passing through two of the given points is equal exactly to the number of all different pairs composed of theseħ points. In particular, every pair of the given 7 points determines uniquely the straight line passing through these points.Ĭonversely, any straight line passing through the pair of the given 7 points determines this pair uniquely because no three of 7 points are on the same straight line.
In fact, this is one of theįirst postulates of the plane geometry, see the lesson Parallel lines under the topic Angles, complementary, supplementary angles in the section Geometry in this site. How many are there different straight lines each passing through two of the given points?įrom Geometry you know that for any two different points in a plane there is one and only one straight line passing through these points. This lesson introduces Combinations, one of the subjects of Combinatorics.Īnother subject of Combinatorics, Permutations, is considered in the lesson Introduction to Permutations.īefore giving the general definitions, let us consider simple examples.Įxample 1You are given 7 different points in a plane such that no three of them are on the same straight line.


 0 kommentar(er)
0 kommentar(er)
